Perturbative experiments are an experimental technique in which a process, here the plasma transport, is actively perturbed to study its dynamical properties. As such this technique allows to separate different transport phenomena in one experiment, which together determine the overall (static) transport.
We systematically optimize the various aspects of perturbative experiments using system identification techniques. The goal is to arrive at a full physics understanding, either through comparison of such measurements with advanced turbulent transport modelling, or by determining the structure of empirical transport models. This allows us to predict and control transport in present-day devices and future experiments and reactors such as ITER and DEMO. Specific applications are:
- Perturbation (input) design by minimizing the Cramer-Rao lower bound/Fisher information matrix for transport models
- Transport model estimation using Maximum Likelihood Estimation and Weighted Least Squares directly in the frequency domain
- Estimating the measurement uncertainties through periodic measurements or for non-periodic measurements through local polynomial methods
- Model validation through statistical tests, multiple experiments, and comparison to full transport models
- Estimation of the distributed parameters or response
- Study of nonlinear properties of frequency response in perturbative transport experiments, and their effect on the linear transport interpretations
- The relation of overall transport properties to small-scale effects such as plasma turbulence
In this project experts from fusion, system identification, distributed systems work together to arrive at a better understanding of transport and to develop control strategies to reduce overall transport losses crucial for arriving at viable fusion power plants.
An example of such a perturbative experiment and its subsequent modelling is the estimation of the diffusion and convection from electron temperature measurements. First, the electron temperature is perturbed using electron cyclotron heating. Next, the subsequent resulting temperature measurements are used to estimate the “local” transport coefficients using Maximum Likelihood Estimation. This results in estimates of the profiles of diffusion and convection including their confidence.
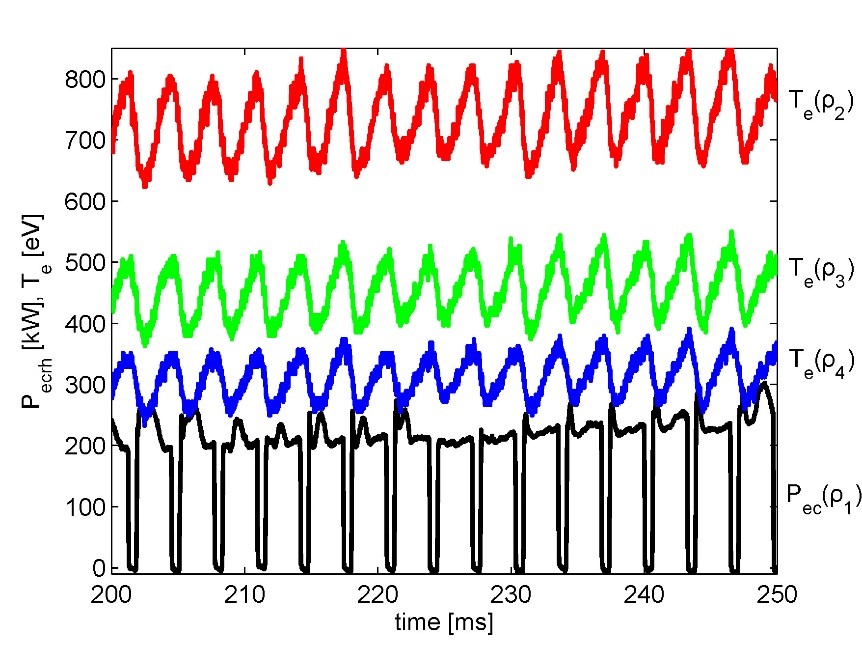
by a heat source (microwave radiation, ECRH) (black) in RTP tokamak, which allows
the distinct estimation of the diffusion coefficient (dispersion) and convectivity (flow)
of the distributed thermal transport.
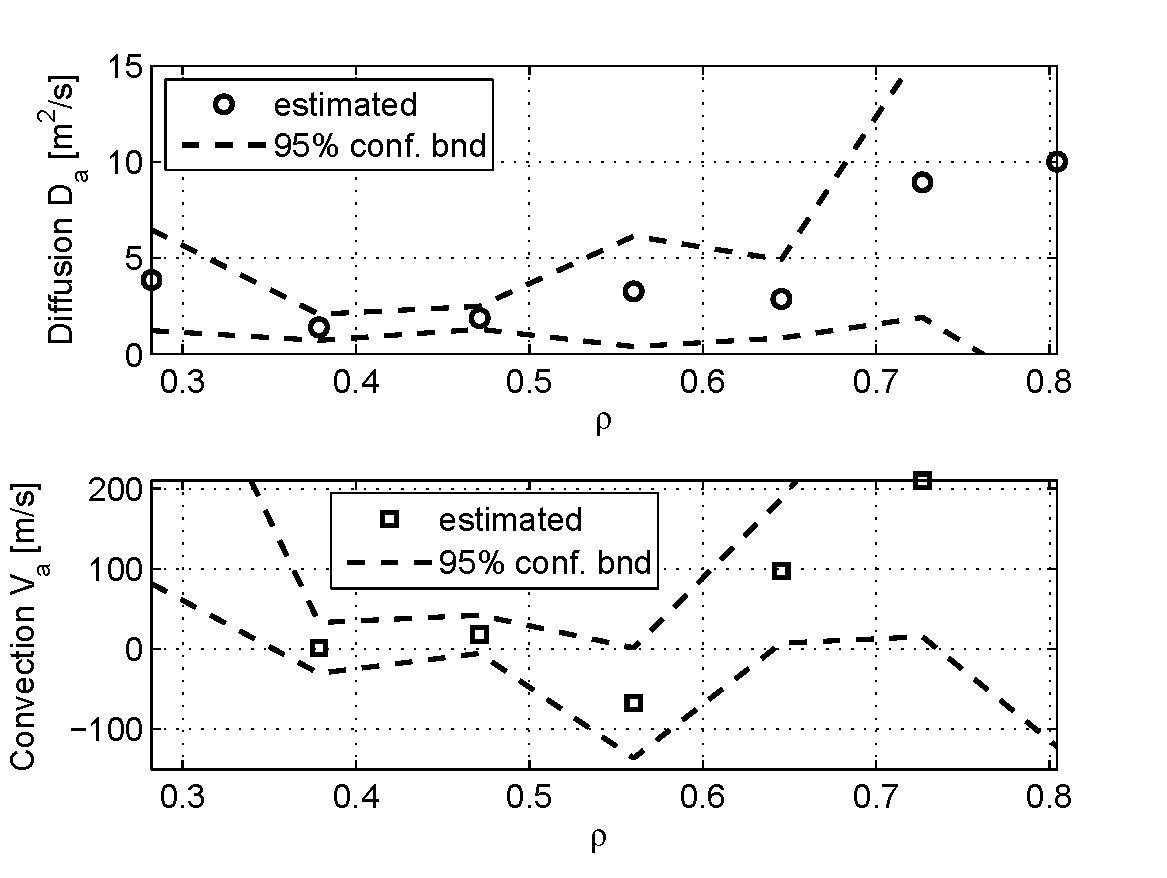
with confidence bounds calculated using the infinite domain maximum likelihood estimator
(RTP-tokamak 19960403.006). The diffusivity estimates are of the correct order, but the convectivity
estimates have a too high uncertainty. (Figure taken from M. van Berkel et al. 2013 IEEE-MSC)